How Many Minifigures?

Hello!

Since this year the LEGO® minifigure is 40 years old, I thought that it might be appropriate if my first technical post focused on something I could explain using minifigures.  And since LEGO® just released Series 18 of their Collectible Minifigures I thought it would be fun to use them for our demonstration today.

Here is the question: If you had a small number of minifigures, how many different figures could you make with them?

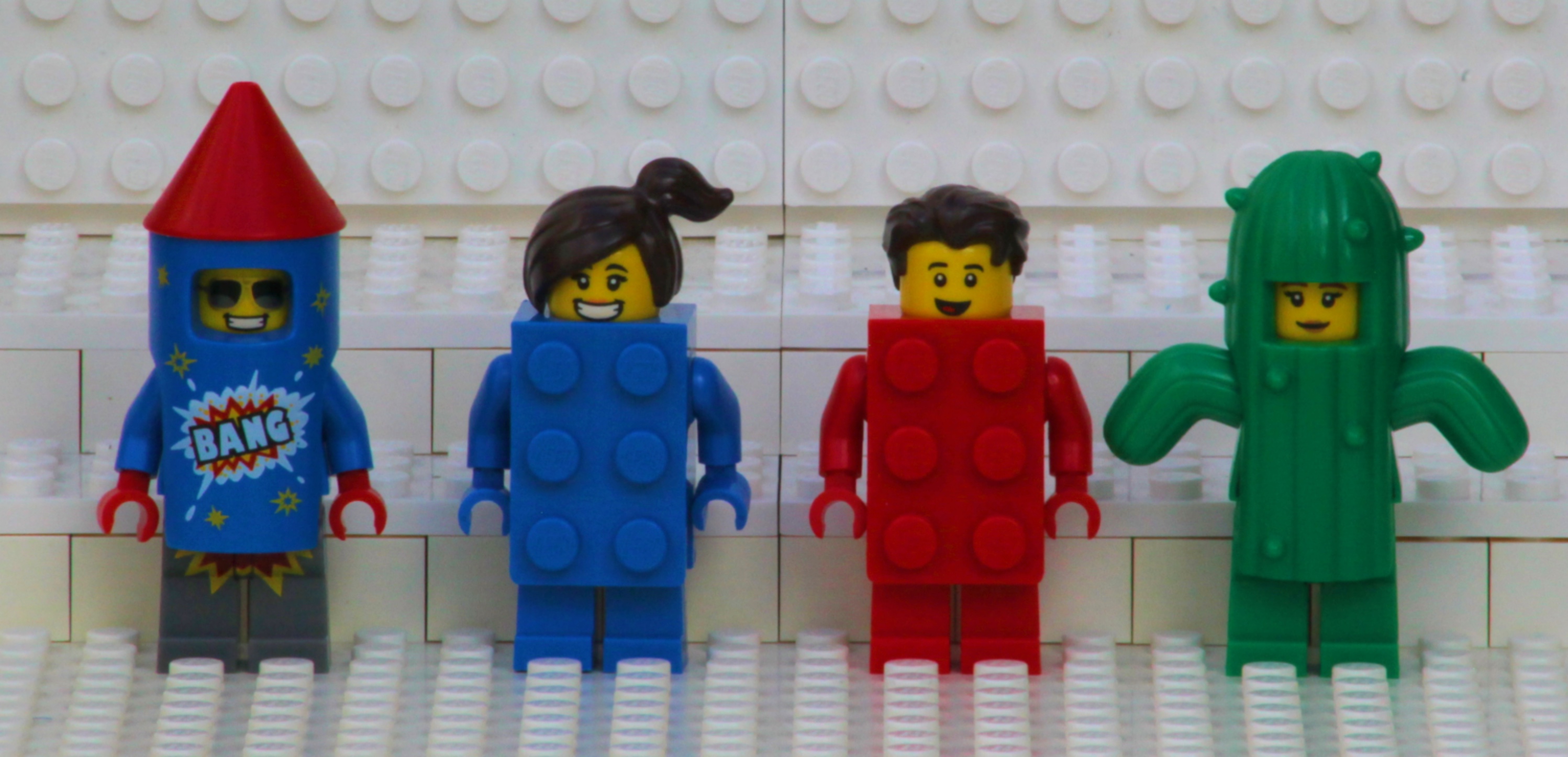
Here is a picture of all the Series 18 minifigures.

There are 17 figures in this set.  Each of these minifigs has a different body, head, hat/hair, and legs.  Most of them have accessories, like the clown’s balloon animals.  And a few of them have parts that attach around the neck or waist of the minifigs, like the Dragon Man’s tail and wings.  To keep things simple we will say that a minifigure is made up of only a body, a head, a hat/hair, and set of legs.  The accessories, waist parts, and neck parts won’t count to making a different figure.

Often in mathematics and science in order to gain a little understanding of the problem, we simplify it by reducing the number of things we are working with.  Then once we think we understand the concept we see if it works for the larger case we are dealing with.  In this case, instead of trying to figure out how many different minifigs we can make with these 17 minifigures, we might want to try to see how many we can make with just two minifigures.  And for this, we will use two of my most favorite minifigures of all time, Brick Boy andBrick Girl!

I am now going to introduce a notation that we can use to label each minifigure so that way we can write out the way a particular minifigure is made without using a lot of words or pictures.  I am going to use four letter “words” to describe a minifigure.  Each letter in order will tell us what original figure the legs, body, head and hair/hat came from.  In this small case, I will use a G to let us know the part came from the Brick Girl, and a B to let us know it came from the Brick Boy.  So the following words GGGG, and BBBB would describe the original minifigures (pictured above).  And GBGB, GGBB and GBBG  would describe the figures in the next picture.  These words are not easy to say aoud (though it would be fun to try), but they make it a lot easier to quickly describe a minifigure, and compare it with another minifigure.

So how do we solve this?  One thing we know in this example is that there are two different choices we can make for the first letter in word.  It can be a G or a B.  So we know we can make at least two different minifigures with either blue or red legs.  We knew this already but it is good to state the obvious when working things out.  We can write these minifigure words like this B*** and G***.  I am using the stars (*’s) as a placeholder for parts of the word we have not yet to decide on.  Next we can look at how many options we have for each of these cases for the next letter.  Again, we have two options (B or G) and we can apply it to both the previous words.  So we now can write BB**, BG**, GB**, and GG**.  Now we know we can make at least four different figures!  Now we might be able to see just how we got here.  We had two options for the first letter, then two options for the second letter.  To get the total for the number of ways we can write the first two letters in the word, we took the number of options of the first letter MULTIPLIED by the number of options of the second letter.  This is great!  Because it gives us a way to guess at how many ways we can write the first three letters.

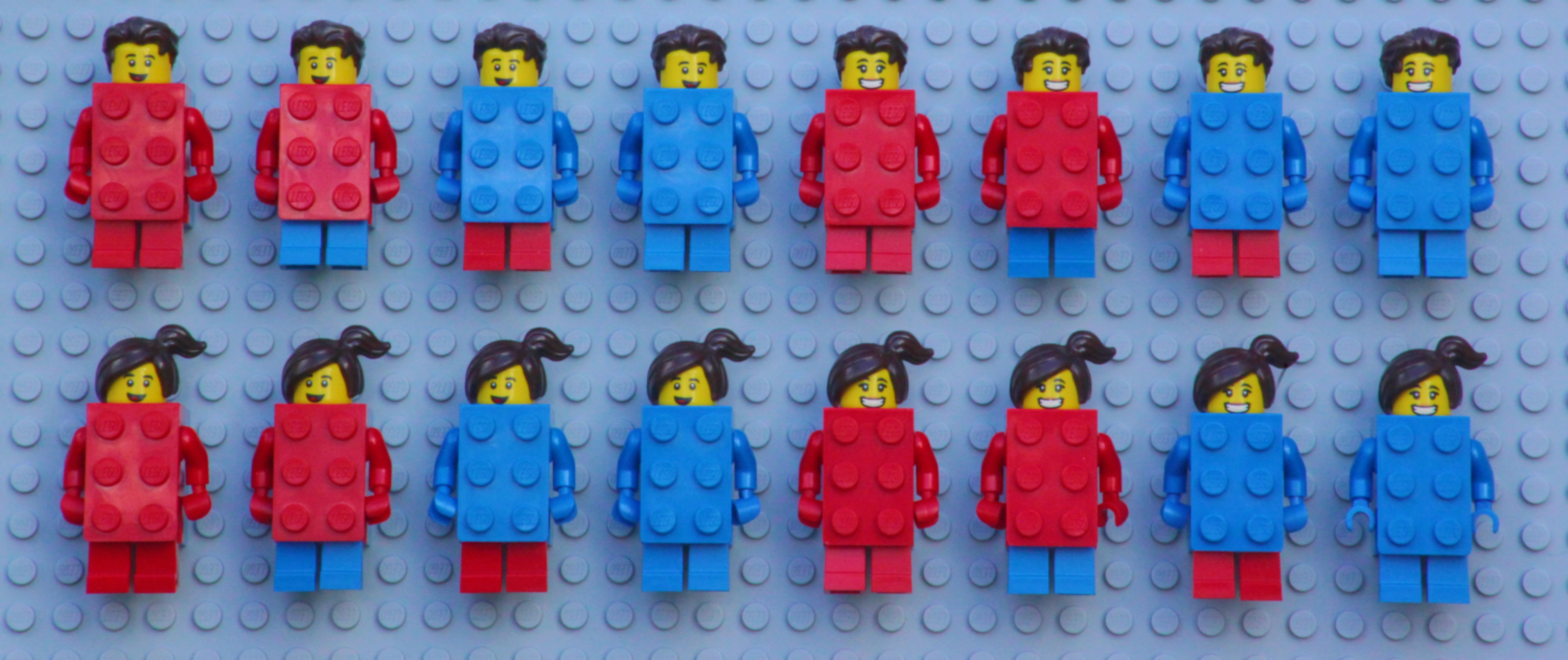
We again have two options for the third letter.  And we know we have four ways to write down the first two letters.  So we might be able to predict that the number of ways to write the first three letters is 4 MULTIPLIED by 2, or eight ways.  And here are the eight ways: BBB*, GBB*, BGB*, BBG*, GGB*, GBG*, BGG*, and GGG*!  Now you might be able to figure out that the total number of ways we can make a minifigure starting with two minifigures.  If you MULTIPLIED 8 by 2 to get 16, then you are right!  The following picture shows ALL the ways that we can combine these two minifigures to make a different one.

Now that we are sure, we know how to count the different number of ways to combine the minifigures, let’s go back to our original question.  In the collection of minifigures, there are 17 of them.  To get the total number of different minifigures we first recognize that we for each body part we have 17 choices to make.  So to find the number of miniatures we can make we would MULTIPLY 17 by 17 by 17 by 17 (this is the same as RAISING 17 TO THE POWER OF 4).  When we do that, we find that we can make 83,521 different minifigs!  WOW!  That is a lot of different figures we can make with such a small number of starting figures.

HOMEWORK!

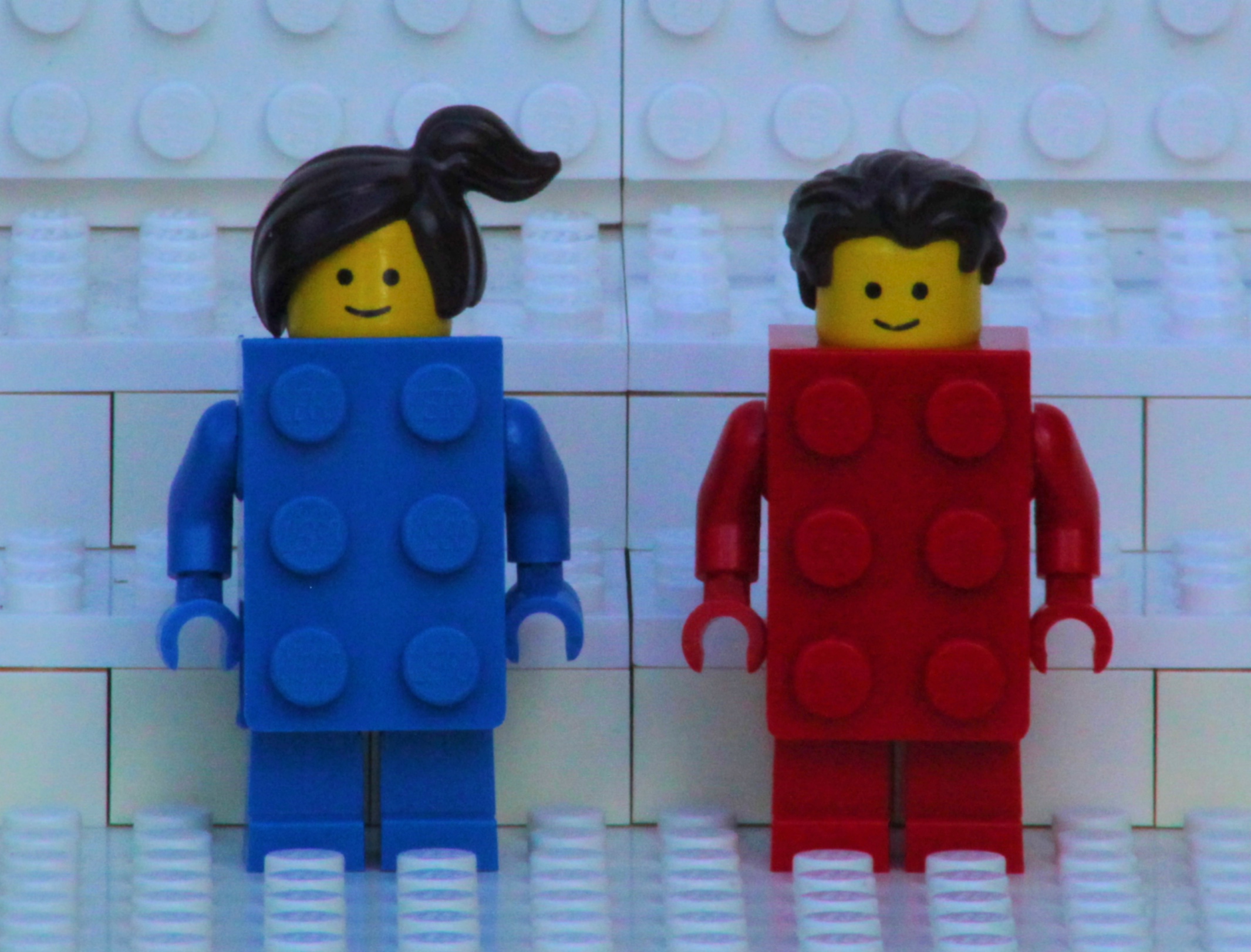
1.  For a long time LEGO® minifigures only had one face, the nice, simple, yellow smiling face.  How many different figures can you make if you have only two starting figures with the same face, and all other parts different?  Kind of like this pair of minifigs.
2. Sometimes minifigures have fun parts that go around their neck, like capes, air-tanks and speech bubbles.  How many minifigures can you make if you had this pair of minifigures and you were able to swap their speech bubble and air-tank?
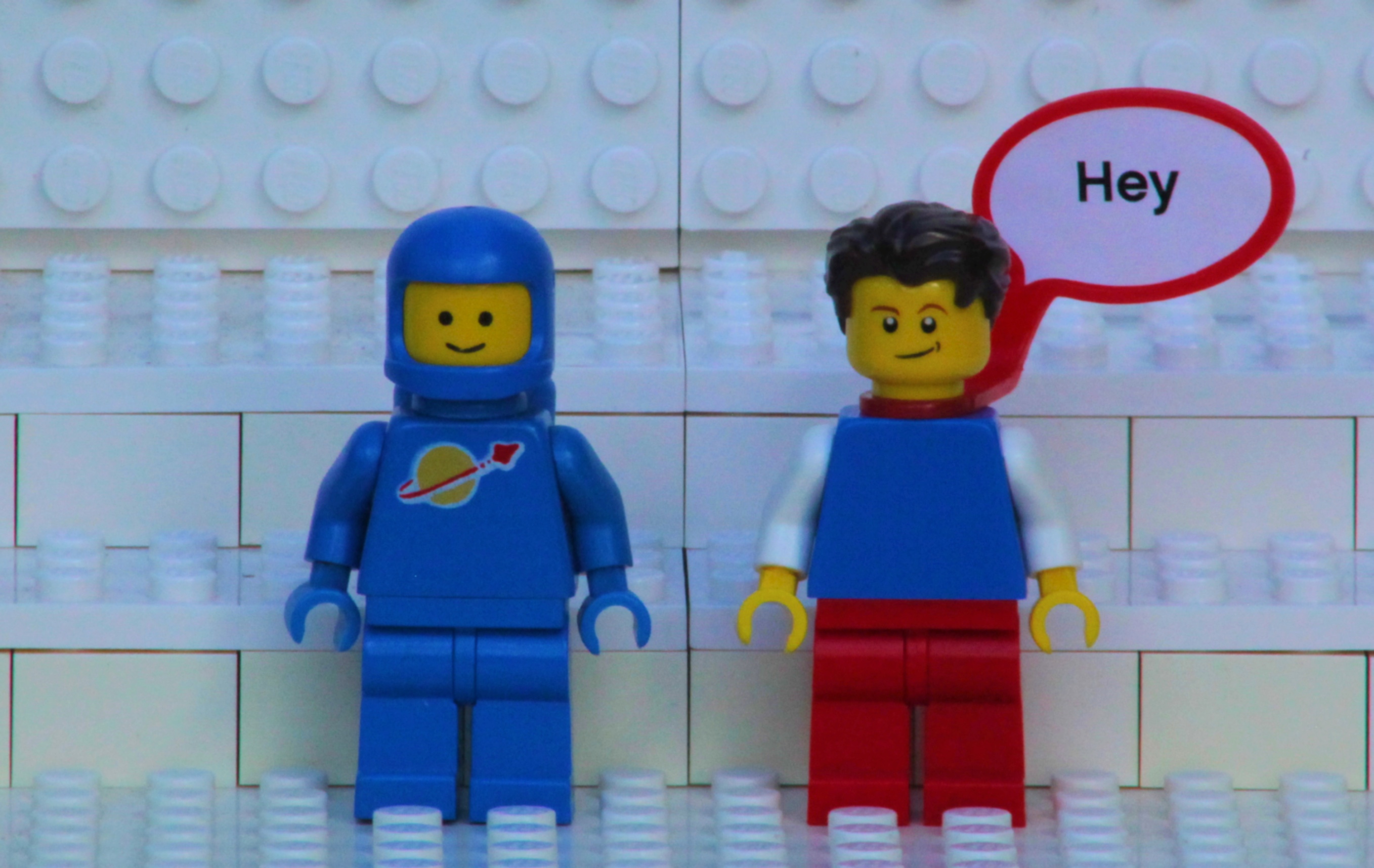
3. With the pair of minifigures from the last problem, what if you wanted the astronaut body to keep the air-tanks, and made the Hey Guy body keep his speech bubble?  How many different figures could you make?
4. In real life some people, like me, are going bald.  What if you had two minifigures like the Boy and Girl minifigure from the example, and you allowed them to be bald sometimes?  How many minifigures could you make then?
5. Sometimes two different pieces that can make up one minifigure cannot be put on the different minifigure at the same time.  For example, from the Series 18 minifigures, the helmet of the Fireworks Guy and Cactus Girl both have hair/hat pieces that cannot be put together with Brick Girl or Boy’s body.  This means that the actual number of minifigures that can be made with this series is less than the 83,521 figures that we calculated above.  Now if those pieces cannot be used together on the same minifigure, then how many minifigures can you really make with the Series 18 minifigures?  NOTE: This question is much more difficult than the previous questions.  So don’t worry too much if you cannot figure it out.